Introduction
Calibration interval analysis has been a popular topic the past few months. From direct questions to LinkedIn discussions, people seem to have a lot of questions regarding calibration interval analysis. As a result, I created a post on the 5 Best Calibration Intervals Guides. Now, I am proposing a new calibration interval analysis method.
After reviewing many of the guides that I recommended, I began to realize most methods available were more complex than simple. I am an engineer with a background in quality control statistics, so it is easy for me. However, most technicians are not engineers and the methods are beyond their mathematical aptitude. Even the military-trained technicians that work for me are not great when it comes to statistics. So, I wanted to develop a method that was simplified to increase time efficiency and allow me to delegate the task of calibration interval analysis to my technicians and administrative personnel.
New Method
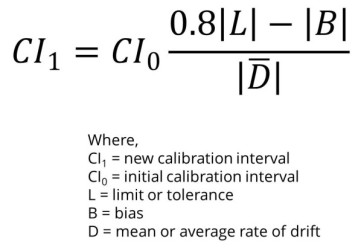
The goal of my proposed equation is to establish a calibration interval that minimizes the occurrence of nonconformance (i.e. out of tolerance) events. The equation uses the calibration limit(tolerance), instrument bias (from the most recent calibration), and average drift rate to predict a calibration interval. The result is an estimated number of months until the instrument is predicted to not conform to specifications.
Furthermore, I have tried to increase confidence in the prediction by adding the ability to reduce the calibration tolerance by a desired percentage. This becomes helpful for those who are trying to conform to ANSI Z540.3-2006 requirements (e.g. risk analysis and acceptance limits), or those who have established corrective action (e.g. adjustments) thresholds.
Testing
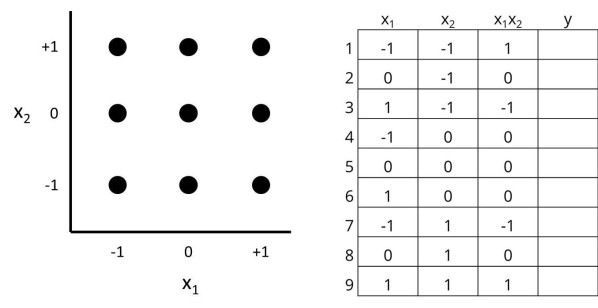
Using instrument adjustment decision rules that I have used in the past, I tested this equation based on 80% of the calibration limit (i.e. p = 0.8). The testing was performed using a Full-Factorial experiment design with two factors at three levels (i.e nk = 32). Without getting too technical, I designed a highly efficient, nine-step experiment that tested two variables at three levels (e.g. high, mid, and low) and evaluated the results in relation to the independent variables and their interactions. However, I still need to perform some qualitative analysis to ensure that this method has not been previously developed and will satisfy quality requirements.
Read more about Full-Factorial Experiment Designs.
http://www.statease.com/pubs/doesimp2excerpt–chap3.pdf
Constraints
After testing the equation under various conditions, I observed scenarios where the equation could be abused or yield bad(inconclusive) results. Therefore, I developed a list of constraints to minimize or eliminate these conditions; but, the constraints could vary slightly based on value of percentage, p.
My proposed list of constraints:
1. Must have 3 years or more of calibration history.
2. The average drift rate must not exceed 50% of tolerance.
3. The bias must not exceed 75% of the tolerance.
4. New calibration intervals must be between 3 and 60 months.
Conclusion
In conclusion, I believe that I have developed a simplified method for performing calibration interval analysis. However, I still have additional analysis and evaluation efforts to perform prior to publishing a paper and presenting my results. By releasing this information, I hope some of you are willing to try this method and give me your feedback.






2 Comments